原文链接: https://leetcode-cn.com/problems/merge-two-sorted-lists

英文原文

You are given the heads of two sorted linked lists list1 and list2.

Merge the two lists in a one sorted list. The list should be made by splicing together the nodes of the first two lists.

Return the head of the merged linked list.

 

Example 1:

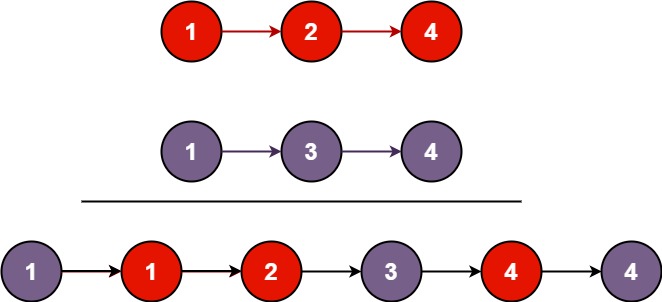
Input: list1 = [1,2,4], list2 = [1,3,4]
Output: [1,1,2,3,4,4]

Example 2:

Input: list1 = [], list2 = []
Output: []

Example 3:

Input: list1 = [], list2 = [0]
Output: [0]

 

Constraints:

• The number of nodes in both lists is in the range [0, 50].
• -100 <= Node.val <= 100
• Both list1 and list2 are sorted in non-decreasing order.

中文题目

将两个升序链表合并为一个新的 升序 链表并返回。新链表是通过拼接给定的两个链表的所有节点组成的。 

 

示例 1：

输入：l1 = [1,2,4], l2 = [1,3,4]
输出：[1,1,2,3,4,4]

示例 2：

输入：l1 = [], l2 = []
输出：[]

示例 3：

输入：l1 = [], l2 = [0]
输出：[0]

 

提示：

• 两个链表的节点数目范围是 [0, 50]
• -100 <= Node.val <= 100
• l1 和 l2 均按 非递减顺序 排列

通过代码

高赞题解

1. 前言

递归解法总是给人一种“只可意会不可言传”的感觉，代码一看就懂，自己动手一写就呆住了，很难受。究其原因，一是我们练习不够，二是理解不够。

2. 什么是递归？

递归的例子在平时生活中很容易见到，比如：

开个玩笑😁

什么是递归呢？函数在运行时调用自己，这个函数就叫递归函数，调用的过程叫做递归。
比如定义函数 $f(x)=x+f(x-1)$：

[]
def f(x):
    return x + f(x-1)

如果代入 $f(2)$：

• 返回 $2+f(1)$；
• 调用 $f(1)$；
• 返回 $1+f(0)$；
• 调用 $f(0)$；
• 返回 $0+f(-1)$
• ……

这时程序会无休止地运行下去，直到崩溃。
如果我们加一个判断语句 x > 0：

[]
def f(x):
    if x > 0:
        return x + f(x-1)
    else:  # f(0) = 0
        return 0

这次计算 $f(2)=2+f(1)=2+1+f(0)=2+1+0=3$。我们从中总结两个规律：

• 递归函数必须要有终止条件，否则会出错；
• 递归函数先不断调用自身，直到遇到终止条件后进行回溯，最终返回答案。

3. 递归解法

根据以上规律考虑本题目：

• 终止条件：当两个链表都为空时，表示我们对链表已合并完成。
• 如何递归：我们判断 l1 和 l2 头结点哪个更小，然后较小结点的 next 指针指向其余结点的合并结果。（调用递归）

<,,,,,,,>

代码

感谢 @huwt 提供的 c++ 代码！

[]
class Solution:
    def mergeTwoLists(self, l1: ListNode, l2: ListNode) -> ListNode:
        if not l1: return l2  # 终止条件，直到两个链表都空
        if not l2: return l1
        if l1.val <= l2.val:  # 递归调用
            l1.next = self.mergeTwoLists(l1.next,l2)
            return l1
        else:
            l2.next = self.mergeTwoLists(l1,l2.next)
            return l2

[]
class Solution {
    public ListNode mergeTwoLists(ListNode l1, ListNode l2) {
        if (l1 == null) {
            return l2;
        }
        else if (l2 == null) {
            return l1;
        }
        else if (l1.val < l2.val) {
            l1.next = mergeTwoLists(l1.next, l2);
            return l1;
        }
        else {
            l2.next = mergeTwoLists(l1, l2.next);
            return l2;
        }

    }
}

[]
class Solution {
public:
    ListNode* mergeTwoLists(ListNode* l1, ListNode* l2) {
        if (l1 == NULL) {
            return l2;
        }
        if (l2 == NULL) {
            return l1;
        }
        if (l1->val <= l2->val) {
            l1->next = mergeTwoLists(l1->next, l2);
            return l1;
        }
        l2->next = mergeTwoLists(l1, l2->next);
        return l2;
    }
};

复杂度分析

如何计算递归的时间复杂度和空间复杂度呢？ 力扣对此进行了 详细介绍 ，其中时间复杂度可以这样计算：

给出一个递归算法，其时间复杂度 ${\mathcal{O}(T)}$ 通常是递归调用的数量（记作 ${R}$） 和计算的时间复杂度的乘积（表示为 ${\mathcal{O}(s)}$）的乘积：${\mathcal{O}(T) = R * \mathcal{O}(s)}$

时间复杂度：${\mathcal{O}}(m + n)$。

$m$，$n$ 为 $l_{1}$ 和 $l_{2}$ 的元素个数。递归函数每次去掉一个元素，直到两个链表都为空，因此需要调用 $R=O(m + n)$ 次。而在递归函数中我们只进行了 next 指针的赋值操作，复杂度为 $\mathcal{O}(1)$，故递归的总时间复杂度为 ${\mathcal{O}(T) = R * \mathcal{O}(1)}={\mathcal{O}}(m + n)$ 。

空间复杂度：${\mathcal{O}}(m + n)$。**

对于递归调用 self.mergeTwoLists()，当它遇到终止条件准备回溯时，已经递归调用了 $m+n$ 次，使用了 $m+n$ 个栈帧，故最后的空间复杂度为 ${\mathcal{O}}(m + n)$。

相关题目

以下是一些基础但很经典的题目，值得我们好好练习：

• 344. 反转字符串
• 面试题 08.06. 汉诺塔问题
• 24. 两两交换链表中的节点
• 104. 二叉树的最大深度

欢迎提供 C++ 代码
如有问题，欢迎讨论~

统计信息

通过次数	提交次数	AC比率
782798	1173070	66.7%

提交历史

提交时间	提交结果	执行时间	内存消耗	语言

相似题目

题目	难度
合并K个升序链表	困难
合并两个有序数组	简单
排序链表	中等
最短单词距离 II	中等