原文链接: https://leetcode-cn.com/problems/kth-smallest-element-in-a-bst

英文原文

Given the root of a binary search tree, and an integer k, return the kth smallest value (1-indexed) of all the values of the nodes in the tree.

 

Example 1:

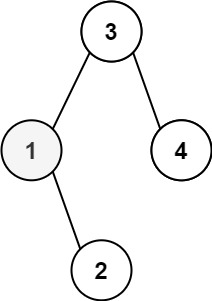
Input: root = [3,1,4,null,2], k = 1
Output: 1

Example 2:

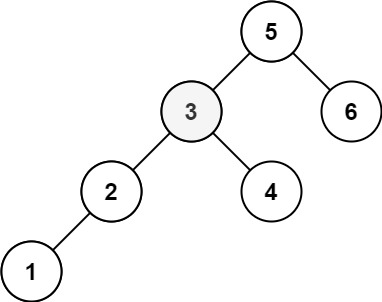
Input: root = [5,3,6,2,4,null,null,1], k = 3
Output: 3

 

Constraints:

• The number of nodes in the tree is n.
• 1 <= k <= n <= 104
• 0 <= Node.val <= 104

 

Follow up: If the BST is modified often (i.e., we can do insert and delete operations) and you need to find the kth smallest frequently, how would you optimize?

中文题目

给定一个二叉搜索树的根节点 root ，和一个整数 k ，请你设计一个算法查找其中第 k 个最小元素（从 1 开始计数）。

 

示例 1：

输入：root = [3,1,4,null,2], k = 1
输出：1

示例 2：

输入：root = [5,3,6,2,4,null,null,1], k = 3
输出：3

 

 

提示：

• 树中的节点数为 n 。
• 1 <= k <= n <= 104
• 0 <= Node.val <= 104

 

进阶：如果二叉搜索树经常被修改（插入/删除操作）并且你需要频繁地查找第 k 小的值，你将如何优化算法？

通过代码

高赞题解

树的遍历 + 排序

朴素的做法是先对二叉树进行一次完整遍历，将所有节点存入列表中，最后对列表排序后返回目标值。

树的遍历可以使用 DFS 或 BFS。

代码：

[]
class Solution {
    List<Integer> list = new ArrayList<>();
    public int kthSmallest(TreeNode root, int k) {
        dfs(root);
        Collections.sort(list);
        return list.get(k - 1);
    }
    void dfs(TreeNode root) {
        if (root == null) return ;
        list.add(root.val);
        dfs(root.left);
        dfs(root.right);
    }
}

• 时间复杂度：树的遍历时间复杂度为 $O(n)$；排序的复杂度为 $O(n\log{n})$。整体复杂度为 $O(n\log{n})$
• 空间复杂度：$O(n)$

---

树的遍历 + 优先队列（堆）

相比于先直接拿到所有节点再排序的解法一，另外一种做法是使用「优先队列（堆）」来做。

由于我们返回的是第 $k$ 小的数，因此我们可以构建一个容量为 $k$ 的大根堆。

根据大根堆的元素个数和当前节点与堆顶元素的关系来分情况讨论：

• 大根堆元素不足 $k$ 个：直接将当前节点值放入大根堆；
• 大根堆元素为 $k$ 个，根据堆顶元素和当前节点值的大小关系进一步分情况讨论：
  • 如果当前节点值元素大于堆顶元素，说明当前节点值不可能在第 $k$ 小的范围内，直接丢弃；
  • 如果当前节点值元素小于堆顶元素，说明当前节点值可能在第 $k$ 小的范围内，先 poll 一个再 add 进去。

树的遍历可以使用 DFS 或 BFS。

代码：

[]
class Solution {
    public int kthSmallest(TreeNode root, int k) {
        PriorityQueue<Integer> q = new PriorityQueue<>((a,b)->b-a);
        Deque<TreeNode> d = new ArrayDeque<>();
        d.addLast(root);
        while (!d.isEmpty()) {
            TreeNode node = d.pollFirst();
            if (q.size() < k) {
                q.add(node.val);
            } else if (q.peek() > node.val) {
                q.poll();
                q.add(node.val);
            }
            if (node.left != null) d.addLast(node.left);
            if (node.right != null) d.addLast(node.right);
        }
        return q.peek();
    }
}

• 时间复杂度：树的遍历时间复杂度为 $O(n)$；使用优先队列（堆）复杂度为 $O(n\log{k})$。整体复杂度为 $O(n\log{k})$
• 空间复杂度：空间多少取决于 d 和 q 使用的容量，q 最多不超过 $k$ 个元素，复杂度为 $O(k)$，d 最多不超过二叉树的一层，复杂度为 $O(n)$。整体复杂度为 $O(n + k)$

---

中序遍历

上述两种节点，都没有利用该树为二叉搜索树的特性。

而我们知道，二叉搜索树的中序遍历是有序的，因此我们只需要对二叉搜索树执行中序遍历，并返回第 $k$ 小的值即可。

不熟悉二叉树的中序遍历的同学，可以看看 （题解）783. 二叉搜索树节点最小距离。

中序遍历有「迭代」和「递归」两种写法。

代码：

[]
class Solution {
    public int kthSmallest(TreeNode root, int k) {
        Deque<TreeNode> d = new ArrayDeque<>();
        while (root != null || !d.isEmpty()) {
            while (root != null) {
                d.addLast(root);
                root = root.left;
            }
            root = d.pollLast();
            if (--k == 0) return root.val;
            root = root.right;
        }
        return -1; // never
    }
}

[]
class Solution {
    int k, ans;
    public int kthSmallest(TreeNode root, int _k) {
        k = _k;
        dfs(root);
        return ans;
    }
    void dfs(TreeNode root) {
        if (root == null || k <= 0) return ;
        dfs(root.left);
        if (--k == 0) ans = root.val;
        dfs(root.right);
    }
}

• 时间复杂度：令 $h$ 为树高，先到达叶子位置（最小节点位置），复杂度为 $O(h)$，然后找到第 $k$ 小的元素，复杂度为 $O(k)$。整体复杂度为 $O(h + k)$
• 空间复杂度：令 $h$ 为树高，复杂度为 $O(h)$

---

最后

如果有帮助到你，请给题解点个赞和收藏，让更多的人看到 ~ (“▔□▔)/

也欢迎你 关注我（公主号后台回复「送书」即可参与长期看题解学算法送实体书活动）或 加入「组队打卡」小群 ，提供写「证明」&「思路」的高质量题解。

所有题解已经加入 刷题指南，欢迎 star 哦 ~

统计信息

通过次数	提交次数	AC比率
170179	226241	75.2%

提交历史

提交时间	提交结果	执行时间	内存消耗	语言

相似题目

题目	难度
二叉树的中序遍历	简单
二叉树中第二小的节点	简单